How Many Triangles Do You See How Many Animals Do You See

There's nothing quite similar a maddening math problem, mind-bending optical illusion, or twisty logic puzzle to halt all productivity in the Pop Mechanics role. We're curious people past nature, but we also collectively share a stubborn insistence that we're correct, dammit, and so we tend to throw work past the wayside whenever nosotros come upon a problem with several seemingly possible solutions.

This triangle encephalon teaser isn't new—shoutout to Popsugar for unearthing it a couple years ago—but based on some shady Cyberspace magic, the tweet beneath reappeared in my feed today and kick-started a new fence on our staff-wide Slack channel, a place traditionally reserved for workshopping ideas, but instead mostly used for yelling about other stuff that we occasionally plow into content.

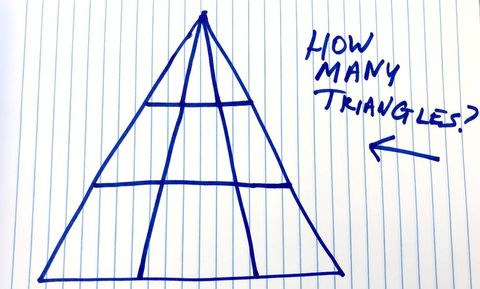
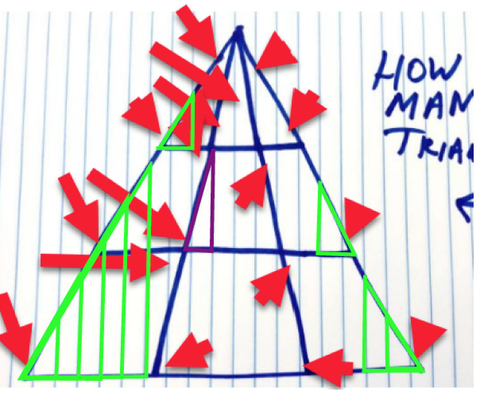
Considering I'm a masochist, I drew the triangle over again and asked everyone on staff to promptly drop what they were doing and effort to solve the simple question: How many triangles can you lot find?

I'll spare yous the full conversation—trust me, nobody wants to see that—but the team'southward responses ranged all over the place. Some editors saw four triangles. Others saw 12. A few saw half dozen, 16, 22. Even more saw xviii. I wiseguy counted the triangles in the A'due south in the question itself, while another seemed to be having an existential crisis: "None of these lines are truly straight, only curves—thus you cannot define any of them as a triangle," he said. "There are no triangles in this photo. Life has no significant."

We then posed the problem to our Instagram followers, whose replies also ran the gamut, from 5 to 14 to 37. While nosotros acknowledge the high probability of trolling here, it's articulate that people respond to the problem many different means.

I could've listened to my colleagues explain their questionable processes all day, only instead, I reached out to several geometry experts to see if we could arrive at a consensus answer. Turns out almost all of the mathematicians I contacted found the aforementioned solution—just not all of them figured it out in the same manner.

If you don't desire to know the reply just yet, terminate reading and try to solve the trouble kickoff. I'll meet you dorsum here when you're done.

Hey, that was quick. Fix for the answer? Unlike some viral math problems that are purposely vague and open for interpretation, this one really does have a slam-dunk, no-doubt-virtually-information technology solution, and it's eighteen. Permit's hear from some of the geometry experts as to why.

"I would approach this just like one approaches any mathematical problem: reduce it and discover structure," says Sylvester Eriksson-Bique, Ph.D., a postdoctoral fellow with the University of California Los Angeles's math department.

The but fashion to course triangles in the figure I drew, Erikkson-Bisque says, is if the top vertex (corner) is part of the triangle. The base of the triangle will then take to be one of the three levels below. "There are 3 levels, and on each you lot tin can choose a base among six dissimilar means. This gives 18, or 3 times half dozen triangles."

Allow'south look at the principal triangle again.

Andrew Daniels

"It'south convenient to generalize to the case where in that location are north lines passing through the top vertex, and p horizontal lines," says Francis Bonahon, Ph.D., a professor of mathematics at the University of Southern California.

In our case, n = 4, and p = 3. Any triangle we notice in the drawing should have one top vertex and two others on the aforementioned horizontal line, and then for each horizontal line, the number of triangles with two vertices on that line is equal to the number of ways we can choose these vertices, Bonahon says—namely the number of ways we can choose 2 distinct points out of north, or "northward cull 2."

Remember high school math? That'southward n(n-1)/ii. And since there are p horizontal lines, says Bonahan, this gives p n(n-i)/2 possible triangles. In our case, that'due south 3x4(four-1)/ 2=xviii.

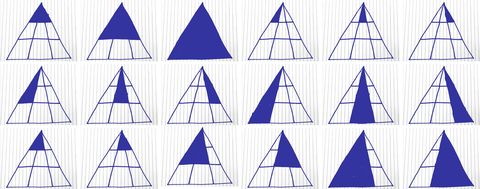
Hither's a handy breakdown of how to find each possible triangle:

Triangle, Triangle, Line, Electric blue, Cone, Sail, Symmetry,

Kory Kennedy

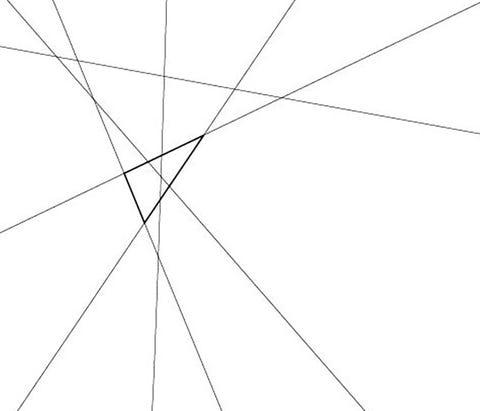
Johanna Mangahas, Ph.D., an assistant math professor at the University at Buffalo, also came to 18—get-go through simple brute-force counting, then through the aforementioned crafty combinatorics every bit to a higher place—but admits our triangle brain teaser isn't quite every bit cool as this one from Po-Shen Loh, Ph.D., a math professor at Carnegie Mellon University in Pittsburgh, as featured in the New York Times last year:

Po-Shen Loh

This one has a slicker mathematical respond, she says, considering here, counting triangles is the same affair as counting combinations of three lines chosen out of six [6-cull-3 = (6*five*iv)/(3*2*1)].

"In that case, every pair of lines intersects and there are no triple-or-more than intersections, and so any choice of three ever gives a triangle," says Mangahas. In the picture I sent her, some lines are parallel, and so they tin can't be part of the same triangle. "If you lot took the same seven lines and shook them up a bit, probabilistically they'd most probable state similar [Loh's] problem and you'd take more triangles and a similar cute respond." (For the tape: 35.)

Whew. I haven't shared this new triangle problem with my coworkers yet. Simply it'southward only a thing of time before they notice it—and argue some more.

🚨 IMPORTANT UPDATE one/30/xx 🚨: Since publishing this story, many, many readers have reached out to let me know that while 18 is indeed an acceptable respond to this trouble, it isn't the only one, due to some unintentional oversight on my part. I could have made this much easier on readers—and, crucially, much easier on my inbox—had I but sketched the triangle on plain, white calculator paper. Merely no.

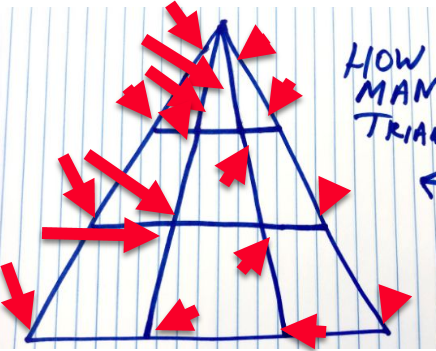
I unfortunately drew this triangle on lined paper, and lots of smart people have correctly pointed out that, well, actually, if yous count the light bluish parallel lines in the image in add-on to the dark blue lines written in marker, there are actually more than 18 full triangles here—considerably more. I never specified to merely use those dark blue lines, and thus, I am wrong. You are right.

I reader, Ralph Linsangan, totally owned me by sending this image, in which he marks every additional triangle found under the technicality, flagging 17 boosted triangles for a total of 35. Behold:

Line, Text, Font, Parallel, Triangle, Graphics,

Ralph Linsangan

That kind of dedication is just one of many reasons I dear Pop Mechanics readers. Nosotros can't go anything past you guys. Until the next teaser!

🚨 However ANOTHER TRIANGLE UPDATE i/31/20🚨: Since posting the last update, I've heard from even more of you, standing to admonish me—and your fellow readers—for not considering additional possible triangles. Allow's hear from reader Derek Schneider, who sent in another graphic suggesting there are 45 triangles.

If we follow the original rules however, I count and boosted 9 that are definite (in green) and one that could be open to interpretation depending on how you visually place the pinnacle vertex (in majestic)…I would personally count it.

Line, Text, Font, Graphics, Parallel, Slope, Triangle, Graphic design,

Derek Schneider

Reader Poingly, meanwhile, wrote in to say we've been making a "grave error" in counting the triangles all along:

Accept the bottom correct corner, for instance, it shows ane arrow for one triangle. However, these light blue lines could conceivably form as many equally 3 triangles in this one corner alone:

Poingly

While some of these MAY be somewhat debatable (ie, where EXACTLY exercise the light blue lines intersect the dark ones and practise they technically class a triangle or a quadrilateral), I have counted Vii Boosted triangles that may be made in this way. This bring the total number of triangles up to 42.
The bad news is that nosotros missed some triangles. The skilful news is that this confirms that life clearly DOES accept meaning, every bit evidenced by the exact number: 42.

Outstanding point, Poingly. Reader James Goodrich took it another step further, suggesting we open our minds to consider what a triangle could be:

Well, co-ordinate to your reader, who pointed out 17 boosted triangles (using the "Andrew didn't specify what lines tin incorporate the 3 edges of a triangle" clause), failed to conspicuously find quite a lot more than. Take, for example, the bottom-left mini-triangle in the 30 January 2020 "Important Update" addendum. Would not the areas of the mini-triangle and the area of the rhombus adjacent to it, combined, make for some other triangle?

Another idea for consideration: Triangles have iii angles (who would have guessed?); however, I would postulate that how y'all draw a triangle, by style of said angles, would generate different triangles. Given a triangle T, with vertices A, B, and C, t-i might indeed be described by ABC, with B being the fundamental angle. I suggest that t-ii, being described past BAC, is dissimilar. Similarly for BCA.

If we and then take a particular example, right-angle triangles, we tin can derive sine, cosine, and tangent functions (SOH, CAH, TOA). If we were to apply that to the triangle (and relax the right-bending requirement, information technology might hateful that BAC is different than CAB. Of course, exceptions are fabricated for isoscolese and equilateral triangles (the latter would just have 3 singled-out triangle definitions).

I haven't quite thought of how to quantify each suggestion (and applying the latter afterward the erstwhile would increase the count notwithstanding), so I don't have an easy number for y'all to use in an updated important update (if you found my ideas worthwhile to update).

I did, James. And I'll be waiting. Begrudgingly, I decided to take 1 last stab at figuring out just how many boosted triangles in that location could be given our new chaotic rules, and arrived at 43, for a total of 61:

Line, Triangle, Font, Parallel, Drawing,

Andrew Daniels

I'm quite certain, however, that someone reading this will very chop-chop tell me I'chiliad wrong yet again and deliver proof of even more hidden triangles, sending me down another rabbit hole on the long and winding path to eventual insanity. (Side note: I haven't seen my married woman in three days. Please tell her I love her.) So I'one thousand issuing ane last challenge: If you can detect the most possible triangles in the original paradigm, show me your piece of work, and definitively testify your supremacy, I will update this story one concluding time and crown you the Triangle Rex or Queen, now and forever. Godspeed.